2024-01-23 Lagrangian Mechanics

Newtonian Mechanics:

• Based on force
• Newtons 3 laws

$${\vec{F}}_{\text{net}}=\frac{d\vec{p}}{dt},m\ddot{x}=F_{\text{net}}$$

Lagrangian Mechanics

• Energy based
• Practical problems with Newtonian mechanics led to development of Lagrangian mechanics 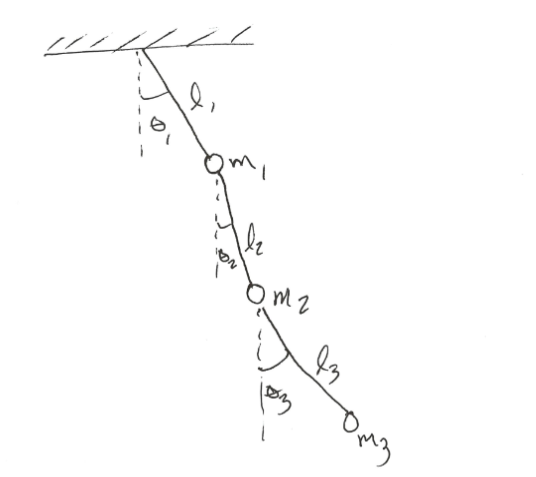Example of problem that would be nearly impossible to solve with newtonian mechanics

The Lagrangian is defined as:

$$L=T-U$$ $$L=L(x,\dot{x},t)$$

It is a function of position, velocity and time

Lagrange’s equation of motion

aka Euler-Lagrange Equation

$$\frac{d}{dt}\frac{\partial L}{\partial \dot{x}}-\frac{\partial L}{\partial x}=0$$

5 simple steps to Lagrangian mechanics

1. Find the kinetic energy (T) $T=\frac{1}{2}m{v}^2$
2. Find the potential energy $U=mgh$ for gravity
3. Calculate Lagrangian $L=T-U$
4. Calculate Euler-Lagrange Equation $\frac{d}{dt}\frac{\partial L}{\partial \dot{x}}-\frac{\partial L}{\partial x}=0$
5. Solve Diff. eq (optional)

Free particle example

Kinetic Energy:

$$T=\frac{1}{2}m{v}^2$$

Potential energy $=0$ or $u_0$

$$L=\frac{1}{2}m{\dot{x}}^2-u_0$$

Take derivatives

$$\frac{\partial L}{\partial \dot{x}}=m\dot{x}$$ $$\frac{\partial L}{\partial x}=0$$ $$\frac{d}{dt}\frac{\partial L}{\partial \dot{x}}=\frac{d}{dt}(m\dot{x})=m\ddot{x}$$

Apply Euler-Lagrange

$$\frac{d}{dt}\frac{\partial L}{\partial \dot{x}}-\frac{\partial L}{\partial x}=0$$ $$m\ddot{x}-0=0⟹m\ddot{x}=0\&\ddot{x}=0$$

Same approach

$$\ddot{x}=0$$ $$\frac{dv}{dt}=0\to v=\text{constant}=v_0$$ $$v=\frac{dx}{dt}=v_0\to x=v_{0}t+x_0$$